CBCI Strategy – Burtis

The following blog reflects a big departure from how I have done things in the past! Maybe old dogs can learn new tricks. After going through the readings for class, I was compelled to look at how I go about teaching. The Foxtrot cartoon below the web really struck a chord. So these lessons start with word problems. Those are usually left for the end, and students dread them. I figure by making them part of the discovery problem, maybe the connection between the math and its applications might be easier to see. If it demystifies word problems and helps the students to understand their value, that’s even better. Every topic in the entire chapter utilizes word problems, so it can be extended to the entire chapter, not just this snippet of it. The other thing I decided was to stress simplifying. In fact, the original draft of the unit web below had “Simplifying Systems of Equations” at it’s center. Isn’t that how we achieve the initial goal of  “turning two equations with two variables into one equation with one variable”? Isn’t solving for a variable just simplifying an equation by isolating the variable? Until we get to 3 x 3 equations and introduce matrix solutions, every other process we utilize in this chapter is already firmly in their tool box, they just need to use them a bit differently…

Applying CBCI to Chapter on Systems of Equations 

The overall unit on Systems of equations has 6 sections and is mapped out at 13-14 days. We break it into 3 smaller units. The first, the one described in this post, covers two equations in two variables and should be about 5 to 6 days.  A section on Linear Programming comes next and is then followed by 3 x 3 systems. Below is a web of the first unit using the first 4 steps from the text. The CBCI components for the two proposed lesson plans to accompany this unit, generalizations, guiding questions, critical content, and key skills, are spelled out in the lesson plans themselves. The web was provided as an overall look at the section.

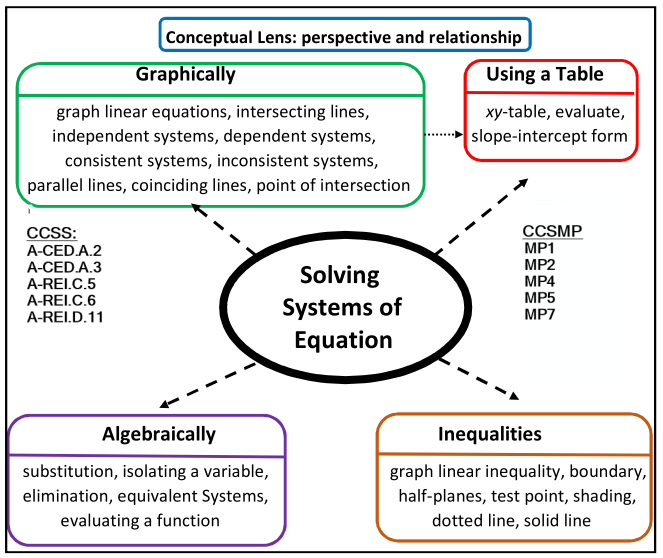
Recommended Pacing:

• Day 1 – Introduction, graphing, tables
• Day 2 – Substitution
• Day 3 – Elimination
• Day 4 – Systems without unique solutions
• Day 5 – Inequalities

This cartoon is an illustration of the disconnect between the math and its application that a lot of student experience, most without realizing it!

 

CBCI Lesson Plan #1 – Burtis

Unit Title: Solving Systems of Equations                 Instructor: Chris Burtis

Subject: Algebra 2                                                          Grade Level: 11 & 12

Lesson Number: 1                                                       Lesson Time Frame: 1 class period

Lesson Opening:

Many situations in the real world involve more than one variable and more than one equation. These functions can be represented in multiple ways. In this unit, we are going to look at how we can solve these systems of equations by using existing knowledge and some creative simplifying. You have the tools, we will look at a slightly different way to use them.

In this opening lesson, we are going to focus on a graphing approach to finding the solutions to a system of equations. We will answer the question: “what is the solution to a system of equations?”.  We will discover the types of answers that are possible, and along the way find a second way to find the solution. At the end of the lesson you will be able to solve a system of equations in two variables by graphing and by using a table. You will also be able to classify a system.

Generalizations:

Students will understand that…

A)  To solve a system of equation, find a set of values that replace the variables and make each equation true.

            Guided Questions for A:

1. What is the relationship between the variables and the solution in a single two-variable equation?
2. Does that same relationship apply to systems of two equations?

B)  A point of intersection of the graphs of the functions is the solution of the system.

           Guided Questions for B:

1. How can you use a graph to solve a system of linear equations?
2. Will the numbers and types of solutions vary?

C)  If graphing by using a table, you may be able to find the solution without creating the graph.

         Guided Questions for C:

1. When making an xy-table, what is the goal?
2. How is it possible to find the solution from the table?

 

Materials/Resources:

• Word problem examples to project on the board for discussion and discovery at each step.
• White board to record student thinking.
• Homework handout. (A mix of straight problems and a few word problems)

Assessments:

1. Formative assessment: Observe student’s participation in group and class discussion.
2. A homework assignment where the students will use their newly developed skills to solve by graphing and by using a table.
3. There will be a unit quiz and then a chapter test where these skills will be assessed.

In-Class Process:

Put the students in groups to start.

1. Use a whole-class Chalk Talk (adapted to them giving the teacher words or ideas they recall) to guide discussion of systems of linear equations (this is NOT a new concept, as it was discussed in Algebra 1). Then ask the groups to discuss the first two guided questions, jotting down their thoughts. After 2-3 minutes, bring to whole class discussion to have them share out their thoughts, culminating in the concept of the solution.
2. Project the first word problem on the board (for concept B). In their small groups, take 5 minutes to answer the second set of two guided questions. The whole class discussion will need to include the information of classifications.  Hopefully they will recognize the special cases, teacher just needs to provide the name for the classifications. If this came up in the Chalk Talk, great. After discussing their thinking, solve the problem on the board in whole class.
3. Project the second word problem on the board (for concept C). This time, in their groups, ask them to consider the last two guided question, and then to create tables in anticipation of graphing, but not to graph. Bring back together and share out their thoughts, which hopefully leads to them finding the point of intersection by identifying matching ordered pairs between the two tables.
4. Put a system of equations on the board (this can be without a word problem) and ask the groups to solve the problem. It is one that does not have an integer answer, so exact answers by graph or table will not be possible…The discussion then leads them to the fact that in the next lesson they will start looking at algebraic solutions.
5. Homework will be 9 problems, 5 asking them to solve by graphing and 3 by table. (Make at least 3 of them word problems.) The last question will be a short answer question asking:”Can you think of a way to classify the system without graphing or making a table?”.

 WORD PROBLEM FOR B. (#2)

You can choose between two tennis courts at two city parks to play tennis. One park charges $4 per hour. The other park charges $2 per hour plus a one-time fee of $4. How many hours must you play for the cost to be the same?

 WORD PROBLEM FOR C. (#3)

There are 25 bikes and trikes at the park. The bikes and trikes have 60 wheels in all. How many bikes and trikes are in the park?

SYSTEM FOR PART 4

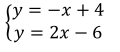
Teacher notes: Depending on the level of your students, the process of turning the word problem into equations can be included in the groups, or can be done whole class before groups start talking. They will have had plenty of practice doing this skill in chapters 1 and 2, so just depends on where you want them to spend their time. The same applies to converting from standard form to slope-intercept. If you feel your students need more practice on either or both of those skills, break the lesson into 2 parts! Section 1 should be quick, since this is a review of Algebra 1 topic, so groups there is optional. In Section 2, depending on recall from Algebra 1 and who their teacher was, they may already be familiar with the classifications, and simply asking them to define the terms may suffice. Section 4 has another option. If teaching a college prep or honors class, teacher can ask the groups to create a system that is not solvable by graphing or tables. I do not teach either of those, so I chose to give them a problem and have them determine why it can’t be solved via the two options we talked about.  If time becomes an issue, start day 2 with #4, since it is the segue to algebraic methods anyway. As a suggestion, before moving into group phase I would project the guided question up on the board so they can refer to it easily.

Below are links for anyone needing more examples of solving by graphing:

https://www.youtube.com/watch?v=T1-yEF6ZBGA

https://www.youtube.com/watch?v=iMyowM_cPww

https://www.youtube.com/watch?v=AVm3eAVGi7M

These are links to explain the classification of systems:

https://www.youtube.com/watch?v=wtLGDhVoxvs

https://www.youtube.com/watch?v=x70Id8sIDL0

Here is a link to solve by using tables:

https://www.youtube.com/watch?v=BtNWyGSYEX0

CBCI Lesson Plan #2 – Burtis

Unit Title: Solving Systems of Equations                Instructor: Chris Burtis

Subject: Algebra 2                                                         Grade Level: 11 & 12

Lesson Number: 3                                                       Lesson Time Frame: 1 class period

Lesson Opening:

We have now discovered three methods for serving systems of equation; graphing, using tables, and substitution. The substitution method was our first algebraic version. To work, the systems needed at least one equation already solved for x or y, or an equation that could easily be converted. We are not always that lucky, so we need an algebraic Plan B. Today we are going to learn about the elimination method.

Generalizations:

Students will understand that…

A)  A system with both equations in standard form and having matching coefficients on either variable can be solved using elimination.

Guided Questions:

1. What was our major goal with our first step in substitution?
2. Can you accomplish the same goal with these equations?
3. Is there any similarity in this method and substitution?

B)  An equivalent system can be used to solve a system with two equations in standard form but without any matching variables.

Guided Questions:

1. Is there a way to make corresponding coefficients match?
2. How will you perform the last step in this case?

For Problem #3, still the same concept…

1. How can I get corresponding coefficients?
2. Is there more than one way to do this problem?

 

Materials/Resources:

• Word problem examples to project on the board for discussion and discovery at each step.
• White board to record student thinking.
• Homework handout. (A mix of direct problems and word problems)

Assessments:

1. Formative assessment: Observe student’s participation in group and class discussion.
2. A homework assignment where the students will use their newly developed skills to solve by elimination.
3. There will be a unit quiz and then a chapter test, where these skills will be covered.
4. Whole class discussion of Headlines created by the groups.
5. Possible Extension: Start class on Day 5 asking the students to take 15-20 minutes and answer the question “Compare and Contrast Substitution and Elimination”

In-Class Process:

Put the students in groups to start.

1. Project the first word problem on the board (for concept A). In group, discuss what is different from yesterday (substitution) and pose the first three Guided questions. After 5-10 minutes, (Teacher can determine time as he/she wanders the room) Back to whole class to share thoughts and ideas. Use whole class to share solution
2. Project the second problem on the board (for concept B). In their small groups, take 5 minutes to answer the second set of two guided questions. Back to whole class to share out. After discussing their thinking, solve the problem on the board in whole class.
3. Project the third word problem on the board (extension of B). This time, in their groups, ask them to consider the last two guided question. Small group for 5 minutes and then back to whole class to share thinking. Solve in whole class scenario
4. Wrap-up the lesson by having the students, in groups, write a Headline about what they learned in class.
5. Homework will be 10 problems, 4 with matching coefficients, 3 needing one equation multiplied, and 3 needing both equations multiplied. I would make at least 4 of them word problems.

Word Problem for A. (#1)

The senior class at HHS and BHS planned trips to a Reds game. HHS filled 7 vans and 5 buses with 171 students. BHS filled 12 vans and 5 buses with 211 students. Each van and each bus carried the same number of student. How many students can a van carry? How many students can a bus carry?  

Word Problem for B. (#2)

A student took 60 minutes to answer 20 questions on a test. There were multiple-choice questions and extended-response questions. She took 2 minutes to answer each multiple-choice question and 6 minutes to answer each extended-response question. How many of each type of question were there?

Word Problem for C. (#3)

The school that Michael attends is selling tickets to a play. On the first day of ticket sales, the school sold 7 adult tickets and 6 student tickets for a total of $111. The school took in $146 on the second day by selling 10 adult tickets and 7 student tickets. What is the price each of one adult ticket and one student ticket?

Teacher notes: Depending on the level of your students, the process of turning the word problem into equations can be included in the groups, or can be done whole class before groups start talking. They will have had plenty of practice doing this skill in chapters 1 and 2, so just depends on where you want them to spend their time.  The Headlines exercise in #4 depends on time availability and if you have used the Headline thinking routine and students are familiar with it. If no time, an exit ticket is always an option, and the Headline routine can be backed up to start the next class with. The Possible Extension is a direct result of our district initiative to try to improve student’s writing skills. We are required to submit them monthly and this is a nice opportunity to work it into the flow of the unit.

Below are links for solving using Elimination:

https://www.youtube.com/watch?v=z1hz8-Kri1E&spfreload=10   (subtract)

https://www.youtube.com/watch?v=lQEmOB_QzZA  (multiply one)

https://www.youtube.com/watch?v=BqfWgF4nOko  (multiply both)